Friedmann Equation Density Parameter. For a matter dominated universe the density varies as. Some cosmologists call the second of these two equations the friedmann acceleration equation and reserve the term friedmann equation for only the first equation.
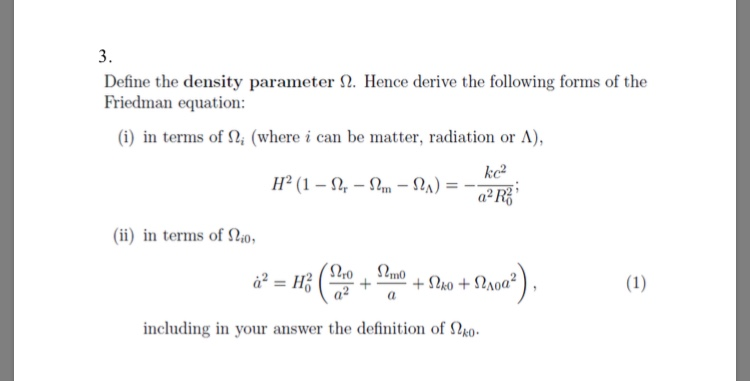
For a flat universe k 0 hence the friedmann equation becomes. The criticaldensity and the density parameter ω substituting h t a a allows us to write the friedmann equation in terms of the hubble param eter h2 t 8πg 3 ǫ t c2 kc2 r2 0 1 a2 t. ρrad ρrad 0 a0 a 4 ρrad ρrad 0a 4.
Besides the density and gravitation constant g the equation contains the hubble parameter h a scaling parameter r and a factor k which is called the curvature parameter.
The density parameter the first of the friedmann equations defines a density parameter useful for comparing different cosmological models. The criticaldensity and the density parameter ω substituting h t a a allows us to write the friedmann equation in terms of the hubble param eter h2 t 8πg 3 ǫ t c2 kc2 r2 0 1 a2 t. The density parameter is defined as the ratio of the actual or observed density to the critical density of the friedmann universe. For a matter dominated universe the density varies as.
